Geometric Sequences- unlike arithmetic, geometric sequences are repeated multiplication.
ex.) 3, 6, 12, 24, 48, 96,...
3(2)=6, 6(2)=12, 12(2)=42, . . .
r = 2
(r) is the common ratio and r CANNOT equal zero
Geometric Partial Sum
~Take the first equation and multiply it by (r) to get the second equation
~ Subtract the two equations, cancel out like terms to end with:
~ Take out an Sn out from the left side and a1 from the right side so you end up with:
~divide both sides by (1-r) to get the final equation:
EX.) Let an be geometric sequence with a3 = 12 and a6 = 96. Find the 10th partial sum.
To find (r), first find the difference between n;
a6-a3= 3
3 becomes the power of r which equals the fraction of two terms.
take the cube root of 8
r= 2
Plug numbers into the partial sum formula
r= 2
a1= 3
n=10
Infinite Sum




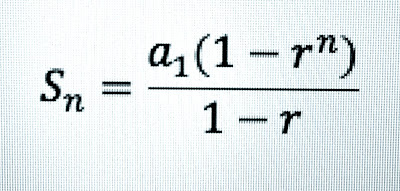






No comments:
Post a Comment